← The Nuclear Atom

Rutherford Scattering

Saturday, February 5, 2022

Rutherford's Observations

While observing the scattering of alpha-particles in atoms, Rutherford realized the equally-distributed positive charge Thomson proposed was unlikely. Instead, Rutherford proposed a small region (called the nucleus) or an atom where the positive charge is.

Repulsive force

For a projectile of charge $ze$, the nucleus hypothesis would predict a repulsive force as such:

$$F=\frac{1}{4\pi\epsilon_0}\frac{\left|q_1\right|\left|q_2\right|}{r^2}=\frac{\left(ze\right)\left(Ze\right)}{4\pi\epsilon_0r^2}$$

With the nucleus theory, particles always experience the full positive charge of the atom, unlike in the Thomson model.

Scattering

Particle projectiles follow hyperbolic paths passing by the nucleus of atoms, according to the following equation in polar coordinates:

$$\frac{1}{r}=\frac{1}{b}\sin{\phi}+\frac{zZe^2}{8\pi\epsilon_0b^2K}\left(\cos{\phi}-1\right)$$

Since the particle begins at $\phi=0$ and $r\rightarrow\infty$ and the final position is $\phi=\pi-\theta$ and $r\rightarrow\infty$, the previous equation can be reduced to

$$b=\frac{zZe^2}{8\pi\epsilon_0K}\cot{\frac{1}{2}\theta}=\frac{zZ}{2K}\frac{e^2}{4\pi\epsilon_0}\cot{\frac{1}{2}\theta}$$

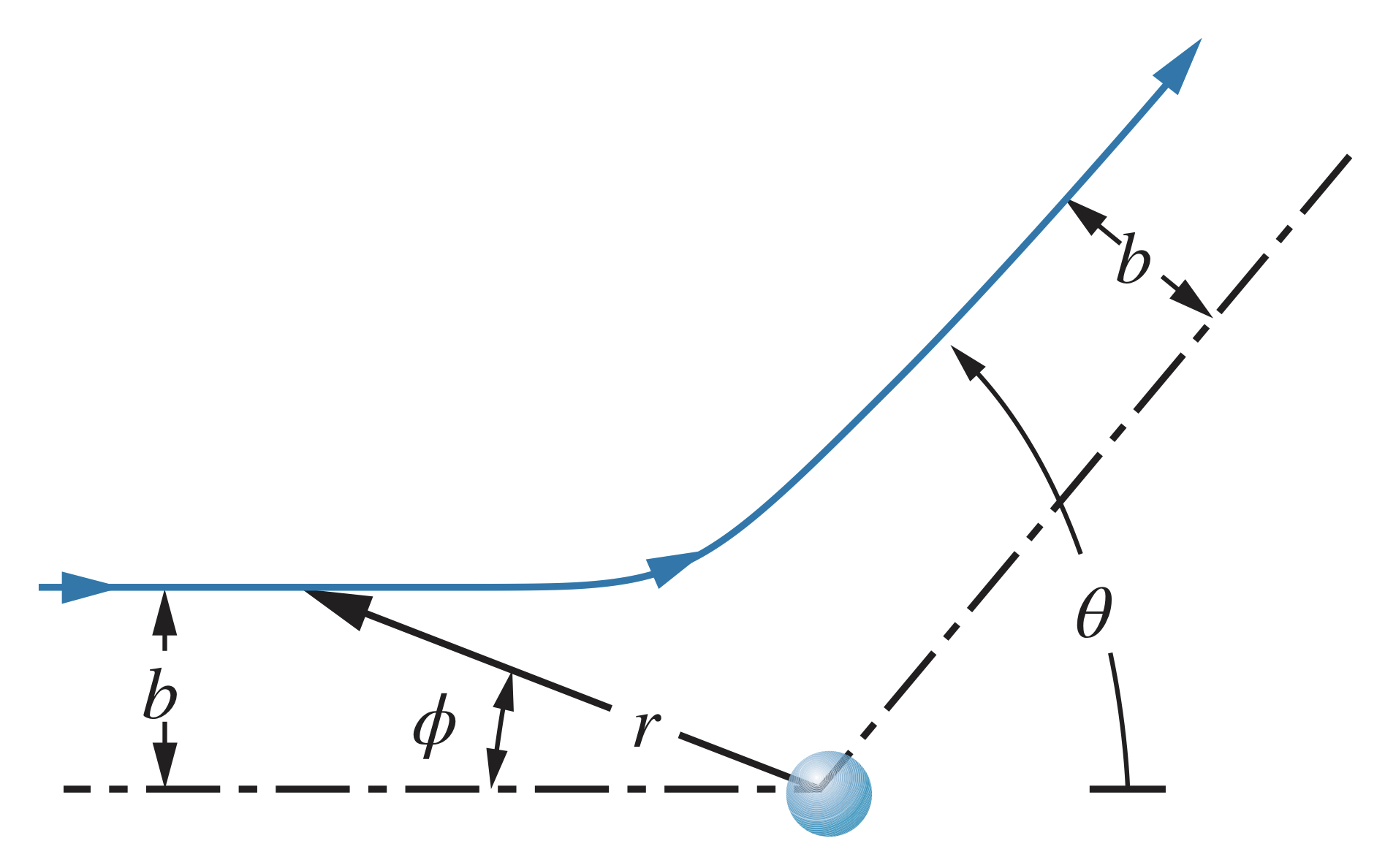
The constant term $e^2/4\pi\epsilon_0$ is equal to $1.44$ eV * nm.

The larger the distance $b$, the less the particle will be scattered. The closer the particle comes to the nucleus (smaller $b$), the more it will be scattered.

Rutherford Scattering

Some projectiles scatter at angles greater than $\theta$

For a foil of $N$ atoms, one atom thick, the total area is $N\pi R^2$. For the scattering to be greater than $\theta$, the impact parameter must be between $0$ and $b$.

Therefore, the probability of the projectile falling within that range is $\pi b^2/\pi R^2$.

For foils greater than one atom thick, say $t$ thickness with $A$ area, $\rho$ density, and $M$ molar mass, the volume of the foil is $At$, the mass $\rho At$, and number of moles $\rho At/M$. The number of atoms (and therefore nuclei) per unit volume is

$$n=N_A\frac{\rho At}{M}\frac{1}{At}=\frac{N_A\rho}{M}$$

where $N_A$ is Avogadro's number. Using this, the fraction of projectiles scattered at angles of less than $\theta$ is

$$f_{\lt b}=f_{\gt \theta}=nt\pi b^2$$

Experimental verification

To find the fraction of particles scattered within a small range $\theta$ (between $\theta$ and $\theta + d\theta$), the impact parameter must be between $b$ and $b + db$. The fraction, $df$, is then

$$df=nt\left(2\pi b~db\right)$$

Using the previous equation for $b$, however, $db$ can be calculated to be

$$db=\frac{d}{d\theta}\left(\frac{zZ}{2K}\frac{e^2}{4\pi\epsilon_0}\cot{\frac{1}{2}\theta}\right)=\frac{zZ}{2K}\frac{e^2}{4\pi\epsilon_0}\left(-\csc^2{\frac{1}{2}\theta}\right)\left(\frac{1}{2}~d\theta\right)$$

Therefore,

$$\left|df\right|=\pi nt\left(\frac{zZ}{2K}\right)^2\left(\frac{e^2}{4\pi\epsilon_0}\right)^2\csc^2{\frac{1}{2}\theta}\cot{\frac{1}{2}\theta}~d\theta$$

The Rutherford scattering formula, which is the probability per unit area for scattering into the ring, is

$$N\left(\theta\right)\frac{\left|df\right|}{dA}=\frac{nt}{4r^2}\left(\frac{zZ}{2K}\right)^2\left(\frac{e^2}{4\pi\epsilon_0}\right)^2\frac{1}{\sin^4{\frac{1}{2}\theta}}$$

Closest approach to the nucleus

As a projectile approaches the nucleus, its kinetic energy is transformed into electrostatic potential energy since

$$U=\frac{1}{r\pi\epsilon_0}\frac{q_1q_2}{r}=\frac{1}{4\pi\epsilon_0}\frac{zZe^2}{r}$$

The maximum potential energy occurs when the kinetic energy is lowest (since energy is conserved), which occurs at a distance of $r_{min}$ and velocity of $v_{min}$:

$$E=\frac{1}{2}mv_{min}^2+\frac{1}{4\pi\epsilon_0}\frac{zZe^2}{r_{min}}=\frac{1}{2}mv^2$$

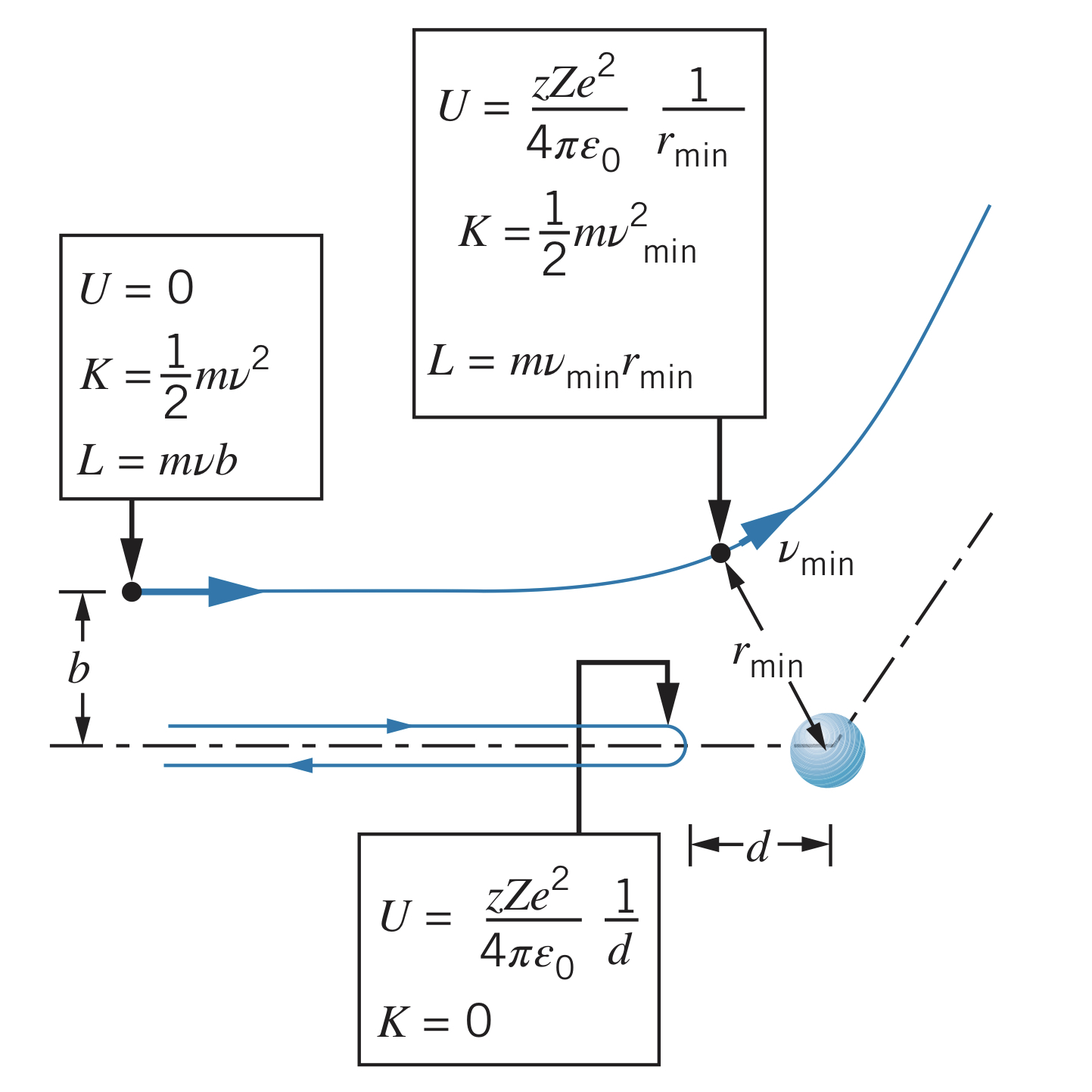
Since angular momentum, $L$, is also conserved, the angular momentum is

$$L=mvb=mv_{min}r_{min}$$

Therefore, $v_{min}=bv/r_{min}$, so

$$\frac{1}{2}mv^2=\frac{1}{2}m\left(\frac{b^2v^2}{r_{min}^2}\right)+\frac{1}{4\pi\epsilon_0}\frac{zZe^2}{r_{min}}$$

Since the kinetic energy is not $0$ at $r_{min}$ unless $b = 0$, the closest approach, $d$, is

$$d=\frac{1}{4\pi\epsilon_0}\frac{zZe^2}{K}$$

The Rutherford scattering law predicts a very small radius for the minimum distance, but it is not always greater than the nuclear radius for large values of $K$ or low values of $Z$. In that case, the particle no longer experiences the full force of the nucleus and so the Rutherford scattering law does not apply.